Why does a cyclist lean when turning?

You might be tempted to assume leaning is the source of circular motion. Cyclist’s lean because of the dynamics of turning rather than to execute the turn. A lean shifts the CyclistCycle Center of Gravity, thereby changing the dynamics of the turn, but not being the source of the turning motion.

Consider what would happen if you didn’t lean? You understand it instinctively. If you tried to remain erect while turning, you would fall over in a direction opposite to that which you are trying to turn.

By now, we should understand the inertial state of a CyclistCycle is to continue in a straightline at constant velocity. When you are turning, you are trying to make the cycle do something it does not want to do. As you turn, the cycle and your body will want to continue moving in the straightline and it is for that reason you lean in the opposite direction to maintain your balance in the turn. And we have already given a name to this inertial tendency, Centrifugal Force.

How does Centrifugal Force manifest itself in turning?

We know that Centrifugal Force is not a true force and could be better named, perhaps calling it an Inertial Force. However it is what it is. In cycling, the effect of Centrifugal Force is to want to maintain straightline motion. However, its actual effect is to create a torque on the cyclist which cause them to fall over in a direction away from the turning circle.

How does a cyclist counter Centrifugal Force?

When a cyclist leans to the inside of the curve, they are creating a counter balancing torque that wants to tip the cycle in the opposite direction to that of the Centrifugal Force. In other words, the lean “balances” out for the cycle’s inertial tendency to want to continue in a straight line.

Computing the lean angle

How much you need to lean to balance the Intertial Tendency is not an option. It is a function of the Centripetal parameters defining the curve: mass, radius, and speed. The cyclist instintively searches for the right amount lean, rather than picking out some arbitrary value. There is an equation that provides the lean angle as a function of these parameters and it is fairly straightforward to compute.

We have three things going on which we need to model:

1. The Centripetal Force generated by the tires “biting” into the road and the road pushing back.  It acts on the CyclistCycle Center of Gravity forcing it to move in the circle.
2. The Centrifugal Force arising in reaction to the Centripetal Force which is really is a way of representing the CyclistCycle inertial motion. It manifests itself in a torque the Cyclist feels as a tipping motion away from the curve.
3. We have the weight of the CyclistCycle which is shifted due to leaning thereby creating a counter torque to balance the Centrifugal Force torque.

The following diagram is one frequently used in explaining how these two torques balance each other out.

 

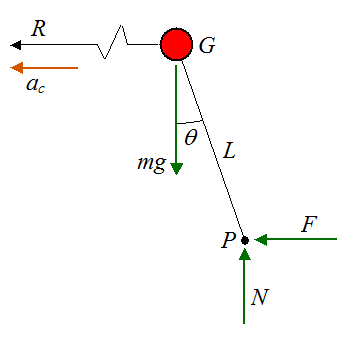
Centripetal Force Components

For anything moving in a circle, we know there must be a Centripetal Force component equal to

F_centripetal = mv²/R

In the diagram, G is the Center of Gravity and R is the circle radius. The Centripetal Force is denoted by F and generated at the tires but the road reaction to the tires “biting.”

Torque 1: Centrifugal Force Torque

 

We know the magnitude of the inertial force to maintain a straight line has a magnitude equal to the Centripetal, is in the opposite direction to the Centripetal Force, and is experienced at the Center of Gravity.

F_Centrifugal = – F_centripetal = – mv²/R

P is the pivot point located at the tires. L is the “lever” distance, and  θ is the lean angle. We know the Centrifugal Force acts on the Center of Gravity in a direction parallel to the ground independent of the lean. So to compute the force creating the torque, we need the component perpendicular to the lean. This gives us

T_Centrifugal = (mv²/R) * Cos Θ * L

Torque 2: Lean Torque

We know that if we were riding slowly in a straightline and leaned to one side or the other, we would fall over. This is the source of the counter balancing torque created by shifting the Center of Gravity. Again we are interested in the component of our weight that is acting perpendicular to the lean plane.

T_Lean = (mg) * Sin Θ * L

Determining the lean angle

The two torques must balance each other out so they must be equal.

T_Lean = T_Centrifugal

  (mg) * Sin Θ * L = (mv²/R) * Cos Θ * L

Tan Θ = (v²/Rg)

We now have a simple expression for the lean anagle as a function of the turn radius, cycling speed, and gravitational constant.

Next Topic:   Lean Angles