Cycling and Rotational Dynamics

Rotational Dynamics in Mechanics is about objects rotating about an axis. You might not think that this applies to cycling but it does in several critical ways.

Pedaling

First, pedaling is about moving pedals around in a circle at some rotational rate called cadence. Its ultimate objective is to move the Crankwheel so that it will pull on the chain. Force is applied at the pedal and creates a turning force at the Crank on the Crankwheel.

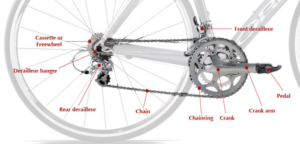
Cycle Drivetrain

The cycle drivetrain transforms pedaling power into the forward power where the rear tire meets the road. This is accomplished by a combination of force and rotational processes. The pedaling process is the front-end or input to the the drivetrain and both involve rotational processes.

Spinning Wheels

The spinning cycle wheels were long thought to be the source of bicycle stability through their gyroscopic moments. Bicycle stability has since been shown to be the result of multiple effects which have made it an ongoing challenge of theoreticians. It may be hard to conceive that in an age of Quantum Field Theory and Gauge Invariant Theories, that bicycle stability was still being worked on, but that is the case.

One important aspect of spinning wheels is they enable the cycle to roll rather than slide over the service. Hockey is a sport where players can slide over the service, but that would simply not work for cycling. The net effect is the Coefficient of Rolling Resistance is an order of magnitude smaller that the Coefficient of Sliding Resistance.

Cycling Balance

A cycle is in contact with the road through its tires. However, the cyclist and the Center of Gravity is located several feet above that point of contact. Simply put, cycles are inherently unstable.

As long as forces are acting longitudinally, that is effectively in straightlines, there is not danger the cycle will fall over. But, when forces are acting laterally, that is counter to the motion, the danger is very real.

One of the challenges in turning is maintaining the balance necessary to counter the effective force of the Center of Gravity wanting to pull the CyclistCycle in the direction of its original straight line motion. This is accomplished by the cyclist leaning into the curve, thereby balancing out the tendency of the cycle to want to rotate opposite to the curve.

 

Next Topic:   Torques and Moments